Thanks to all who responded (Bob, David P., Keene, Robert, Tim and Igor). The more respondents there are, the more extensive my follow-up posts tend to be. (I hope you can stand it.)
                                  |
| ||||
| XGID=-a-a-BC-C-AAdC---c-db---B-:0:0:1:64:0:0:3:0:10 | |||||
21$-51$-31N-32C-64 ..[P p1]"<=62 | |||||
| 1. | Rollout1 | 13/7 11/7 | eq: +0.112 | |||
| ||||||
| 2. | Rollout1 | 10/4 8/4 | eq: +0.111 (-0.001) | |||
| ||||||
| 1 62208 Games rolled with Variance Reduction. Dice Seed: 91243072 Moves: 3-ply, cube decisions: XG Roller | ||||||
For the rollout of the position above (#50 from the Nactation tutorial), making the 7pt is tied with making the 4pt, where both moves keep exactly one spare on the 6pt. This sort of result suggests, in the face of what seems to be a generally prevailing 4pt preference, that actually the 7pt (better for prime) and 4pt (better for board) are roughly equal in value "on average." In choosing which of these two points to make, midpoint/6pt distribution is commonly the key determinant.
In the early game, we typically deal with more checkers on the midpoint and/or 6pt. As most experts intuitively understand, the first spare (especially) and second spare on the 6pt are generally more useful than their counterparts on the midpoint. By contrast (factoring out the difference in pipcount), the THIRD spare on the midpoint is generally more valuable than the third spare on the 6pt. This is because it is more difficult to unstack checkers from the 6pt than from the flexible midpoint. Midpoint checkers are freer to start vacant points (in the outer board), as doing so is much less likely to leave direct shots.
With this principle in mind, let's have a look at Problems #1 and #2 below.
[To see diagrams side by side, widen your window or zoom out using Ctrl-.]
       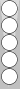            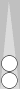               |
| ||||
| XGID=-b----E-C---eE---c-e----B-:0:0:1:00:0:0:1:0:10 | |||||
Backgammon: Opening 42 = .177, 61 = .150 (10k rollouts) | |||||
       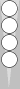            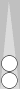               |
| ||||
| XGID=-bb---D-C---dD---c-d---BB-:0:0:1:00:0:0:1:0:10 | |||||
Nackgammon: Opening 42 = .091, 61 = .149 (10k rollouts) | |||||
PROBLEM #1:...In the opening position of Nackgammon, which is a better opening roll: 42 or 61?
I logged XGR++ evaluations for: opening 65 66 and 55 in BG (backgammon, left-hand position), the same rolls in reply to opening 61, then opening 32 33 and 22, and the same rolls in reply to opening 42. Then I noted the counterpart situations for NG (Nackgammon, right-hand position), and compared all twenty-four data points.
For BG, opening 61 gains over 42 by .031 due to blocking larger numbers (vs smaller numbers). Nevertheless, opening 42 beats 61 overall by .027 (again using XGR++evals, for consistency). The primary reason for this .058 difference is the value of unstacking the third 6pt spare instead of the (more flexible) third midpoint spare.
For NG, opening 61 gains over 42 by .052 due to blocking the larger numbers of four back checkers. A comparison of the information imparted in the first sentence of the previous paragraph shows that NG 61 vs 42 represents a greater gain in blocking larger numbers than BG 61 vs 42, by .021.
As NG 61 beats 42 overall by .052, what accounts for the extra .031? I believe it is the fact that the midpoint and 6pt are each reduced by one spare. As discussed earlier, the third midpoint spare is worth more than the third 6pt spare (which should be unstacked), but the second midpoint spare is worth less than the second 6pt spare (which should be kept).
In Nackgammon, the 10k rollout preference for opening 61 over opening 42 by .058 is pretty close to the .052 difference that XGR++ evaluation predicted.
       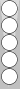            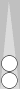               |
| ||||
| XGID=-b----E-C---eE---c-e----B-:0:0:1:00:0:0:1:0:10 | |||||
Standard BG: Opening 42 = .177, 61 = .150 (10k rollouts) | |||||
       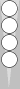   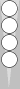                        |
| ||||
| XGID=-b----D-D---eE---d-d----B-:0:0:1:00:0:0:1:0:10 | |||||
Modified BG: Opening 42 = .160, 61 = .180 (10k rollouts) | |||||
PROBLEM #2:... In the modified opening position above (right), which is a better roll: 42 or 61?
In the standard opening position of BG (on the left, above), opening 42 is superior to opening 61 by .020 (eval) or .027 (rollouts). In the modified opening position (on the right), 42 is inferior to 61 by .027 (evals) or .022 (rollouts). The swing is .047 (evals) or .049 (rollouts).
Remember, when comparing NG to BG (after accounting for the effect of larger numbers blocking two extra back checkers), a .031 swing accounted for the lack of both a third 6pt spare and third midpoint spare. Here, in the standard/modified comparison, .049 accounts for the lack of a third 6pt spare while keeping the number of midpoint spares the same. In other words, the Problem 2 comparison is purer: it isolates the effect of removing a third 6pt spare (given that the extra 8pt spare is unloaded with both rolls): it is much more worthwhile to unstack the third 6pt spare (per 42P in standard BG) than the second 6pt spare (per 42P in modified BG).
For those interested in well-played retro-sequences, 52S-52S-32K-1-F-31H-31-21U-54R-54R is a way of transporting both players' 6pt spares back to their respective 8pts (i.e., of reaching the modified opening position diagrammed above). If you want to move back only Blue's 8pt spare, replace 31H with 51H. If you want to move back only White's 8pt spare, replace 32K with 52K. (If you replace both, you will simply re-reach the standard opening position.)
Studying problem #1 and problem #2 will help you make appropriate adjustments to many early game situations with checker distributions that have changed from the opening position, through natural development or otherwise, including the choice of hitting in the outer board versus making an inside point. For example, if you reread Mike's recently posted 51S-62S-42 + variant and Daniel's variants, the rollout results there may suddenly make perfect sense.
                                  |
| ||||
| XGID=-c----C-C---fF---c-c----C-:0:0:1:42:0:0:3:0:10 | |||||
Extra-Mod Opening 42 ...[P S4 U32 Z33 D58] "<=116 | |||||
| 1. | Rollout1 | 8/4 6/4 | eq: +0.069 | |||
| ||||||
| 2. | Rollout1 | 24/20 13/11 | eq: +0.065 (-0.004) | |||
| ||||||
| 3. | Rollout2 | 24/22 24/20 | eq: +0.037 (-0.032) | |||
| ||||||
| 4. | Rollout2 | 24/22 13/9 | eq: +0.036 (-0.033) | |||
| ||||||
| 5. | Rollout2 | 13/11 13/9 | eq: +0.011 (-0.058) | |||
| ||||||
| 1 116640 Games rolled with Variance Reduction. Dice Seed: 25540072 Moves: 3-ply, cube decisions: XG Roller 2 5184 Games rolled with Variance Reduction. Dice Seed: 75619779 Moves: 3-ply, cube decisions: XG Roller | ||||||
PROBLEM #3:... What is the best play and what is the second best play with opening 42 in the extra-modified opening position shown above?
Forty-plus years ago, with a roll of 42, opening pioneer Mike Carson sometimes began his games with the second-best-but-whopper S (Split, 24/20 13/11). Experimentation was the hallmark of the Golden Years.
In a long rollout of the extra-modified opening position diagrammed above, 42S almost overturns 42P. By the B/W error scale, the two plays are "tied."
It is worth noting the comparison of non-P moves to each other. According to rollouts (not shown) of the isolated half moves, 24/20 has the highest equity, and (relatively) 13/9 is -.011, 13/11 is -.023, and 24/22 is -.027. Attempting to predict move strengths by simply combining the half-move values projects a performance of [S U4 D11 Z15], but this is a naive method. In the actual rollout above, the P-less result is [S U28 Z29 D54]. The reason that S and Z gain on U and D is due to the synergy of making a balanced play (one portion up and the other portion down).
Key: P (Point) = 8/4 6/4, S (Split) = 24/20 13/11, Z (reverse split) = 24/22 13/9, U (Up) = 24/22 24/20, D (Down) = 13/11 13/9.
Nactation tutorial • explanation of nacbracs
                                  |
| ||||
| XGID=-b--C-C-B---eE---c-e----B-:0:0:-1:00:0:0:3:0:10 | |||||
Opening 42 .177 "<=10 | |||||
PROBLEM #4:... In a money game, you play opening 42. Your opponent offers you let you play a free deuce from your 6pt to your 4pt (before he rolls). Should you accept the opponent's kind offer?
In the glossary of Backgammon Openings, a "dilly builder" is defined as "A spare in the inner board that is deeper than an unmade point."
With the dilly builder on the 4pt above, Blue's rolls of odd doublets, 33 11 (and 55 if White splits), will clearly be weakened. Moreover, if the remaining 6pt spare is used to hit on or slot any point in the inner board, one can imagine the pain of having the other spare on the 4pt instead of the 6pt, and that loss is greater than the compensating benefit of being able to point on a low point with certain non-doublets or hit from the 4pt in some variations when White splits.
Blue profits surprisingly little from the option of making the 3pt with a subsequent 51. For example (by XGR++ eval), if the opponent replies with 42P, Blue's 51P gains only .004 over 51S (and his 62P is a blunder). Moreover, making the 3pt with 53 suddenly becomes a whopper in most variations!
By 10k rollouts, opening 42P is .177, compared to only .159 with the 6/4 added. In spite of the extra 2 pips gained, the dilliness of Blue's builder reduces his equity by .018.
To further hone your intuition, study the reference position below. D (Down, 13/11 13/8) is tied with the safe-but-dilly N (Near, 13/8 6/4), even though D leaves a direct 6-shot. (If White did not have a second point in her board, D would be clearly better than N.)
Nack
                                  |
| ||||
| XGID=-a--BaD-B---dE-a-b-d--b-B-:0:0:1:52:0:0:3:0:10 | |||||
53P-42P-43S-52 ...[D N5 O29 H45] "<=31*5 | |||||
| 1. | Rollout1 | 13/11 13/8 | eq: -0.277 | |||
| ||||||
| 2. | Rollout1 | 13/8 6/4 | eq: -0.282 (-0.005) | |||
| ||||||
| 3. | Rollout2 | 13/6 | eq: -0.306 (-0.028) | |||
| ||||||
| 4. | Rollout2 | 13/11 6/1* | eq: -0.322 (-0.044) | |||
| ||||||
| 1 31104 Games rolled with Variance Reduction. Dice Seed: 79903269 Moves: 3-ply, cube decisions: XG Roller 2 5184 Games rolled with Variance Reduction. Dice Seed: 79903269 Moves: 3-ply, cube decisions: XG Roller | ||||||