In looking at Phil's first position in the Benjamin thread (diagrammed below), I was amused at how basic it is to comparison-count, even without help from the colorless camp.
Give White double 5s, which is 20 pips, and let her play 18/8(2). Give Blue a 7 and let him play 8/1. This makes the position symmetrical. (20 - 7) = 13. So, Blue is up 13 pips.
But let's pretend for a minute that it's not that easy...
Here is a new pipcount system I call "Twip Count" or just "Twip," where each twip is TWelve pIPs. It's surprisingly simple. You bear off checkers in both directions. (Actually, it is your choice of which direction, but it usually makes sense to bear them off to the nearest tray.)
.....(a) Bearing off to the standard tray, each checker counts as 1 "twip."
.....(b) Bearing off to the midpoint tray(!), each checker counts ZERO.
To minimize actual counting, checkers are first "cancelled" as much as conveniently possible. These cancellations can be either vertical or horizontal. In the first two examples, I'll demonstrate VERTICAL cancelling.
                          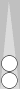        |
| ||||
| XGID=--BBBBBbC---bB-----bbbbba-:0:0:1:00:0:0:3:0:10 | |||||
| What is the comparative count? | |||||
Above is Phil's first position. I request that you visualize the following shift: Move each of the two checkers on White's 18pt, 6 pips in opposite directions. Move one of these checkers to White's 24pt, and the other checker to the midpoint TRAY (which makes it vanish off the board)! When you think you have this visualized, check to see if your vision matches the diagram below.
                                  |
| ||||
| XGID=-aBBBBB-C---bB-----bbbbba-:0:0:1:00:0:0:3:0:10 | |||||
| What is the comparative pipcount? | |||||
Now, vertically CANCEL all symmetrical checkers, ignoring color. If you do it right, all of them will disappear, except for which THREE checkers? When you think you know the answer, scroll to the diagram below.
                                  |
| ||||
| XGID=--------C-----------------:0:0:1:00:0:0:3:0:10 | |||||
15 pips | |||||
Yup, these are the only three checkers that you actually have to count. Count them to the nearest tray, which is to the right -- the midtray (midpoint tray). They look like they're on the 5pt, so count 'em that way, as three on the 5pt. Blue is up 15 pips.
Note that the color of the checkers doesn't matter. The point is that there are three checkers that have progressed 15 pips past the midpoint ridge onto Blue's side of the board (and the other twenty-seven checkers have all been zeroed out). If these three checkers (of any color) were instead on the same point directly above it on the far side, then it is White who would be up 15 pips.
"Wait a minute, Nack! Look at the original diagram. XG tells us that Blue is up only 13 pips, not 15." Good for you, that's observant. Consider, though, these two points:
(1) In the vast majority of positions, your checker play or cube decision is very unlikely to change based on a couple of pips' accuracy in the comparative count. If it does happen to be a position with that degree of sensitivity (usually meaning both a straight race and the relative count being in a small critical range where you'll want to apply a race formula), you can then get a total count for one side (giving you all you need).
(2) In the event that you DO you want an exact Twip count, be aware that when you "bear off" a checker, you're technically moving it to the half-pip ridge just inside the tray rather than to the tray itself. In this example you moved four checkers to the mid-tray (one White checker in the 18pt pre-shift, and the three "5pt" checkers). Those four checkers each moved a half pip less than the rough estimate indicated. Adjusting for those four half-pips, Blue is up only 13 pips (instead of 15). Again, though, that level of detail is rarely necessary.
For our second example, we'll use Phil's (unusual) second position:
                   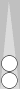  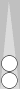             |
| ||||
| XGID=-bBbBbBB-----c----bBBbBbA-:0:0:1:00:0:0:3:0:10 | |||||
| What is the comparative count? | |||||
This is very EASY to count, remembering the irrelevance of color. Move one of the midpoint checkers 11 pips to the blotted 24pt (on the far side). Everything else cancels, so Blue is up 11 pips!
What about the two remaining checkers on the midpoint? Glad you asked. Unless there's a big stack, I tend to ignore midpoint checkers as if they don't exist, because technically they only count 1/2 pip each to the midridge. (Counting them as such gives an exact count of 10.) If I count them in the crude method as 1 pip each, I'll be just as far off (i.e., two half-pips in the other direction, getting a count of 9).
Let's move on to our third example, which is Phil's third position. This would be cumbersome in most pipcount systems.
                                  |
| ||||
| XGID=-bcc--CBBBB-bB---abbB-----:0:0:1:00:0:0:3:0:10 | |||||
| What is the comparative pipcount? | |||||
First, a pre-shift. Imagine that both players (for a fair offset) roll 31 here. I request that you visualize Blue making his 5pt (8/5 6/5), and White anchoring on her 21pt (24/21 22/21). Now verify your visualization with the diagram below.
                                  |
| ||||
| XGID=-acbbBBBABB-bB---abbB-----:0:0:1:00:0:0:3:0:10 | |||||
Now, remove vertically symmetrical checkers | |||||
Now, remembering to ignore color, vertically cancel as much as you can, and see if your vision matches the diagram below.
                                  |
| ||||
| XGID=-acbb----BB---------------:0:0:1:00:0:0:3:0:10 | |||||
Now, horizontally cancel ("reflect") | |||||
Ah, good. Now I can show you an example of horizontally "cancelling," as I promised earlier. This is not a pure cancellation, because there is a count involved. More specifically, I call this method "reflecting."
Reflections are done around the raised bar in the center of the board. Any checker of the left side can be reflected (offset, cancelled) against another checker that is equidistant from the bar on the right side. (6pt vs 7pt, or 5pt vs 8pt, or 4pt vs 9pt, etc.). Any such checker pair (together) counts as 1 twip.
Here, there are four checkers on each side of the bar that can be reflected. The blue ones (obviously), versus the four White ones that are closest to the bar. Count all eight of these checkers together as FOUR twips, make them disappear, and see if your vision matches the next diagram.
                                  |
| ||||
| XGID=-ac-----------------------:0:0:1:00:0:0:3:0:10 | |||||
Count to bear-off tray (and as 1 twip each) | |||||
These four checkers remain. If they were already in the nearest tray (the one to their left), they would count 1 twip each, or 4 twips. How many pips does it take to get to that tray? It's like a bear-off: it takes 7 pips, which I'll round down to half of a twip.
[It helps to remember that moving on Blue's side of the board towards his midpoint is PLUS (meaning a favorable count) for Blue. Moving away from his midpoint is MINUS.]
So, adding the 4 twips we reflected earlier to the 4 twips here, and subtracting the half twip, that means Blue leads by 7 1/2 twips.
Once you have a feel for the size of a twip, you won't need to convert to baby pips. But for now, if you like, multiply by 12. That is, Blue leads by (12 x 7 1/2) = 90 pips.
I recommend going back and reviewing the counts in this post. Remember, the system is new to you and familiarity will increase speed. If to verify you've counted a certain position correctly (or fastest) with Twip, feel free to post it and I'll comment.
                                  |
| ||||
| XGID=-bcc--CBBBB-bB---abbB-----:0:0:1:00:0:0:3:0:10 | |||||
| What is the comparative pipcount? | |||||
Finally, in the Benjamin thread, somebody mentioned another colorless counting system, Coconut. For reference, here is a count of the above position using that system.
With Coconut, you need count only CHECKERS, and only three times! Those counts are in RED. In black is the arithmetic -- the routine arithmetic that you do for every count.
.....First you double the near side men (21 x 2) = 42
.....Add the diagonal (+16 = 58), double again (116)
.....Plus slants (+20 = 136), less 105 (31), times 3 (93)
.....Then shift and cancel (for accuracy)
(Usually, you won't bother with the fourth line, which hones the count from 93 to 91.)
Nack